- English
- Français
- Nederlands
A short explanation of "Le Mystère d'Urbicande"
(Professeur R. de Brok, Brüsel, ?)
By Doctor Jean-Paul Van Bendegem
Translated from Dutch by Eilko Bronsema
Doctor Van Bendegem's contribution is briefly mentionned in Le Guide des Cités, on page 126 of the original edition. We are indebted to Olivier Tissot for the detective work required to trace him back and obtain the permission to reproduce the following article.
On page 19 of the above mentioned book, a mathematical formula is used as an argument against the existence of the Network (Web). The formula of Robick,
Un = (2n+1) + 4(1(2n-1) + 2(2n-3) + … + n1)
is, according to de Brock, mathematicaly incorrect. The development of the right side can be replaced by the summation that gives the simple relation of de Brok:
Un = (2n+1)(2n2+2n+3)/3
The comment by Eugen Robick himself, noted at the side of the page, says that his formula better represents the image of the Network. This can only be interpreted as a reference to the pyramid shape - Robick really meant an octahedron (see further) - of the Network. In this short text I want to express four points:
- The correctness of the equality of the formulas of Robick and de Brok.
- The incorrect rendition of the pyramid on page 18 of the book of de Brok.
- The deduction of the formula of Robick and its relation to the pyramid shape.
- The simple relation between the formula of de Brok and the pyramid shape, which proves that the formula of de Brok is a faithful representation of the phenomenon too.
(1) Let me start with the formula of Robick:
Un = (2n+1) + 4(1(2n-1) + 2(2n-3) + … + n1)
The summation between the brackets on the right side is the part that interests us:
1.(2n-1) + 2.(2n-3)+ … + n.1
The amount of terms in this summation is n, if the summation is between 1 and n. What is the form of a general term for this development? It is a product, of which the first factor is a number i between 1 and n, so 1 < = i < = n and the second factor is 2n - 2i + 1 . So the general term is:
i.(2n - 2i +1) (x)
Let me use ∑ to say that we need to make the summation of the terms like (x) where i is between i and n. Then we can make the formula of Robick a bit simpler:
Un = (2n+1) + 4 . ∑ i(2n - 2i + 1)
In this last formula we can transform i.(2n - 2i + 1) to i.2n - 2i2 + i . Now we can transform the formula and we will find:
∑ i.(2n - 2i + 1) = ∑ i.2n - ∑ 2i2 + ∑ i
The first term is ∑ i.2n . Because in each term we find 2n we can put it outside the summation sign (∑): ∑ i.2n = 2n. ∑ i .
The third term is ∑ i, so we can join these two terms to (2n+1). ∑ i . The value of ∑ i is a known mathematical result:
∑ i = n(n+1)/2
The classic proof is like this:
∑ i = 1 + 2 +3 + … + n -1 + n
∑ i = n + n-1 + n-2 + … + 2 + 1
(switching the terms does not change the summation).
Sum everything:
2. ∑ i = (n+1) + (2+(n-1)+ … + ( (n-1)+2) + (n+1)
At the right side is n times n+1, so:
2. ∑ i = n(n+1) en so i = n(n+1)/2
This leaves us with the ∑ 2.i2 term. Again we can replace the factor 2, so 2 . ∑ i2 . Also this time we find a known result:
∑ i2 = n(n+1)(2n+1)/6
There are several ways to prove that. i2 can be replaced by i(i+1)-i, so ∑ i2 = ∑ i(i+1) - ∑ i. The second term on the right side has already been calculated, so we have to look at i(i+1). If we write out this summation we will find:
1 = 1(1+1)/2
1 2 = 2(2+1)/2
1 2 3 = 3(3+1)/2
…
1 2 3 … n = n(n+1)/2
When we sum everything we will find:
n.1 + (n-2)2 + (n-3)3 + .. + 1.n = ∑ i(i+1)/2
or ∑ i.(n-i+1) = ∑ i(i+1)/2
or 2. ∑ i.n - 2. ∑ i2 + 2.∑ i = ∑ i(i+1)
When we replace ∑ i(i+1) in the above formula we will find:
∑ i2 - 2. ∑ i.n - 2. ∑ i2 + 2. ∑ i - ∑ i
or 3. ∑ i2 = (2n+1) . ∑ i
with help of the last formula we find:
3 . ∑ i2 = (2n+1)n(n+1)/2
which gives the formula:
∑ i2 = n(n+1)(2n+1)/6
When we use these results we can transform the formula of Robick like this:
Un = (2n+1) + 4 .( (2n+1). ∑ i - i . ∑ i2)
Un = (2n+1) + 4. ( n(n+1)(2n+1)/2 - 2n(n+1)(2n+1)/6)
Un = (2n+1)(1 + 2.n(n+1) - 4.n(n+1)/3)
Un = (2n+1)(3 + 6.n(n+1) - 4.n(n+1)/3)
and at last the formula of de Brok:
Un = (2n+1)(2n2+2n+3)/3
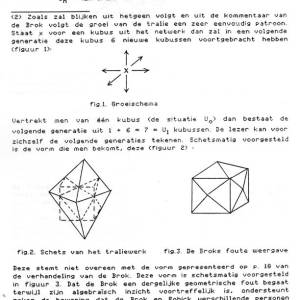
(2) The growth of the cube follows a very simple pattern, as will be demonstrated in what follows, based on the comments by de Brok. If x stands for one cube of the network then the next generation of this cube will be 6 new cubes (fig.1).

If you start with one cube (the situation U0) then the next generation of cubes will be 1 + 6 = 7 = U1 cubes. The reader can draw the next generations by himself. It will become something like this (fig.2).
 fig.2. the network (web) |  fig.3. De Broks' incorrect presentation |
This does not match the figure presented on page 18 of the story by De Brok. This figure is presented as fig. 3. The fact that de Brok made such a geometric error, despite his incredible algebraic insights, suits the theory that de Brok and Robick are two different persons. It isn't unreasonable to say that urbatect Robick has basically a geometric view of the world, and Archivist Isidore Louis is wrong when he claims that both persons must be the same (see L'Archiviste, piece no. 16).
(3) The deduction of the formula of Robick and its relation to the pyramid shape
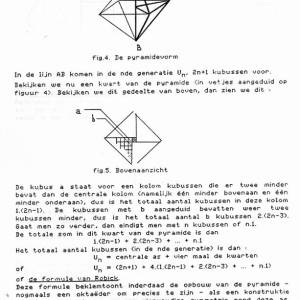
The global shape of a pyramid is as follows (fig.4):

In line AB there will be in the nth generation Un 2n+1 cubes. Then we look at a quarter of the pyramid (the bold lines in figure 4). When we look at this part from above we will see this:
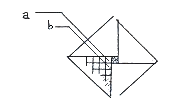
The cube 'a' stands for a column of cubes that has two less than the central column (one less on top and one less at the bottom), so is the total amount of cubes in this column 1.(2n-1). The cubes marked with a 'b' have again two cubes less, so is the total amount of 'b' cubes 2.(2n-3). If you continue like this then you will end up with 'n' cubes of n.1 . The total summation of this quarter of the pyramid is:
1.(2n-1)+2.(2n-3)+…+n.1
The total number of cubes (in the nth generation) will be:
Un = central column + 4 times the quarters or
Un = (2n+1)+ 4.(1.(2n-1)+2.(2n-3)+…+n.1)
or the formula of Robick.
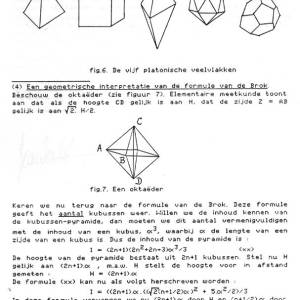
This formula stresses the building of a pyramid, an octahedron to be more precise, as a construction with a central column and four simple symmetric shapes around this column. Please notice that this isn't the only way to describe a pyramid in a pure geometric way. It is very surprising that Robick hasn't noticed that the pyramid-octahedron is one of only five regular Euclidic or Platonic shapes (see figure 6). It cannot be a coincidence that the pyramid is exactly the middle of their progressive scale. It is not the simplest figure but also not the most complex.

(4) The geometric interpretation of the formula of de Brok.
Look at the octahedron (see figure 7). Elementary math shows that if the height CD equals H, then the side Z = AB is equal to √2. H/2.
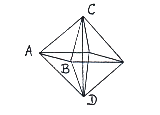
Let us return to the formula of de Brok. This formula shows the number of cubes. If we want to know the content of this cube pyramid then we have to multiply this amount with the content of a cube, α3</supp>, where α is the length of a side of the cube. So the content of the pyramid is: I = (2n+1)(2n<sup>2+2n+3)α3/3 (xx)
The height of the pyramid is 2n+1 cubes. If H equals (2n+1).α, in other words H is the height measured in distance: H = (2n+1).α.
The formula (xx) can be rewritten as follows:
I = ( (2n+1).α.( (√2(n+1/2α))2 + 5.α2/2)/3
In this formula we can exchange (2n+1).α by H and (n+1/2).α by H/2 and √2.H/2 by Z:
I = H.(Z2+5.α2/2)/3
When we estimate a very large number of cubes for a given height H (to get in this way a “classic” pyramid octagon), then will become very small, so in above formula we can skip the 5.2/2 part and keep as the formula for the content:
I = H.Z2/3
This is exactly the formula for the content of an octahedron!
This means that the formula of de Brock is a discrete representation of the formula for the content of an octahedron, where the factor (2n+1) stands for the height and (2n2+2n+3) measures the area of the square the octahedron is build on. So it can hardly denied that the formula of de Brok, like the formula of Robick, gets the essential part of the network (web).
A last small comment: more support for the non-algebraic but geometric insight of Eugen Robick can be found in his comment. He writes “ma formule, en son expansion infinie,…” (page 19). Robick's formula contains a development, but for every generation Un it will be finite.